In May 2009 WP:LAYOUT conducted two studies.[A] The first was to determine the proportion of section titles used in the standard appendices of all articles within the English Wikipedia.[B] The second was to determine the degree of conformity in all articles within the English Wikipedia as defined by WP:LAYOUT.[C] Below are the results.[D]
| Footer type
|
Count
|
Proportion
|
Error bound[iii]
|
| References |
979 |
48.95% |
±2.19%
|
| Notes[i] |
89 |
4.45% |
±1.04%
|
| Bibliography[i] |
35 |
1.75% |
±0.57%
|
| Footnotes |
13 |
.65% |
±0.352%
|
| Sources |
1 |
0.05% |
[ii]
|
| Works cited |
1 |
0.05% |
[ii]
|
| Citations |
0 |
0% |
[ii]
|
- ^ a b Possible confounding
- ^ a b c Sample size too small to calculate reliably
- ^ Error bounds are calculated with a 95% confidence interval[E]
Blocked: Articles with sections
| Category
|
Count
|
Proportion
|
Error[ii]
|
| Single |
114 |
46.53% |
±6.246%
|
| Yes |
93 |
37.959% |
±6.0765%
|
| No |
35 |
14.285% |
±4.3815%
|
| Maybe |
3 |
[i] |
[i]
|
- ^ a b Sample size too small to calculate reliably
- ^ Error bounds are calculated with a 95% confidence interval[E]
Blocked: Applicable by WP:LAYOUT
| Category
|
Count
|
Proportion
|
Error[note 1]
|
| Yes |
93 |
70.992% |
±7.77%
|
| No |
35 |
26.717% |
±7.577%
|
| Maybe |
3 |
[note 2] |
[note 2]
|
- ^ a b Sample size too small to calculate reliably
- ^ Error bounds are calculated with a 95% confidence interval[E]
At this point the author would like to interrupt this list to indicate a possible application of the value attribute found in Template:Cnote2. Very long lists similar to this one may benefited by having it be broken down. Remember to remove the link to an inline occurrence, giving the feel of an regular list, set n=0.
- ^ ChyranandChloe; et al. (5 May 2009). "Standard Appendicies Conformity Statistics". Journal of Wikipedia Layout (in Chinese). San Francisco, United States: Wikimedia Foundation. 1 (1): 3–4. Retrieved 2009-09-19.
- ^ Null hypothesis tests have been omitted.
| Assumption
|
| n > 30
|
| np0 > 10
|
| n(1-p0) > 10
|
| Simple Random Sample
|
- ^ a b c Note that binomial distributions are discrete, these error bounds assume a normal distribution. Calculated as <math>\hat p \pm z^* \sqrt{ \frac{\hat p \left ( 1- \hat p \right )}{n
</math>, distributed as such: 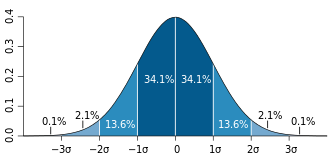 }}
}}
 }}
}}